- Poker Card Combinations Ranking
- Poker Combinations Rankings
- Poker Pairs Ranking
- Poker-hand-ranking-combinations
- Poker Combinations Rank
What Are The Poker Hands
Three of a Kind. Three cards of the same value (e.g., 3, 3, 3; or Jack, Jack, Jack). While this is a lower ranking hand, it's more commonly pulled than flushes or full houses. When you're betting, it's good to remember what hands are most the most common. The Poker Hands. Here’s a ranking chart of the Poker hands. The chart lists the rankings with an example for each ranking. The examples are a good reminder of the definitions. The highest ranking of them all is the royal flush, which consists of 5 consecutive cards in one suit with the highest card being Ace. There is only one such hand in. Texas Holdem Rankings for All 169 Starting Hands. Ever since the early days of Texas holdem poker, players have attempted to analyze and organize the 169 possible two card starting hands found in the game. Use the OFFICIAL poker hand rankings to know what beats what in poker. Download the PDF list of poker hands. When you know that there are 52 cards in play and 2,598,960 possible combinations.
Though the rules vary according to the poker tournament variant, the highest poker hand ranking always wins. Poker hand order is determined by the pattern formed by the cards. If the patterns formed are the same, then the poker card rankings determine the winner. Individual cards in poker order are ranked highest to lowest: A, K, Q, J, 10, 9, 8, 7, 6, 5, 4, 3 and 2.
Suits are not ranked, so hands that differ by suit alone are of equal rank. The top poker hands to play fall into nine categories using a standard deck of 52 cards. A player can form 2,598,960 distinct poker hands since the order in which they are dealt with are inconsequential. Since the suits are of equal rank, a player can form 7462 distinct texas Holdem poker hands.

What Are Poker Suits?
♣ Club
♦ Diamond
♥ Heart
♠ Spade
What Are Top Poker Hands To Play?
1. Pocket Aces - Every poker player knows the power of this poker card ranking. Pocket aces are also the strongest starting hand in poker hand order. They make the best poker hand pre-flop and the odds are 4:1 favourite over any other poker hand. One in every 221 poker hands is a pocket ace and if you're a serial grinder, you should know how to play this best poker hand.
Also known as Pocket Rockets, Two Pips.
2. Pocket Kings - Pocket Kings are a favourite against any hand (except aces). This texas Holdem poker hand is winning two-thirds of the time against Ace high and therefore ranks just below pocket aces in poker hand order in real money poker game.
Also known as Cowboys, King Kong, Ace Magnets.
3. Pocket Queens - One of the best poker hands to play poker strongly pre-flop. You should be raising or re-raising from any position with these Ladies. This texas Holdem poker hand is likely to be called by plenty of opponents with not the best poker hands.
Also known as Ladies.
4. Ace-King Suited - This is a texas Holdem poker hand easy to fall in love with. While pre-flop in remains a great hand beating post pocket pairs (except kings and aces). But it’s important to realize that if you don’t connect with the flop, then you only have an ace-high.
Also known as Big Slick, Anna Kournikova, Big Ugly.
5. Pocket Jacks - This is the best poker hand against any unpaired hand and strong pre-flop over any weaker waits. A great texas Holdem poker hand to play in late position with an unraised pot, but be wary of the early position raises.
Other Nicknames: Brothers, Suckers.
6. Pocket Tens - Pocket tens are a strong starting hand in poker. This texas Holdem poker hand will still win against overcards most times and is good enough not hitting a third on the flop. If the pot has witnessed a lot of action before you, it’s an easy fold with this texas Holdem ranking.
Also known as Dimes, TNT, Dynamite.
7. Ace-Queen Suited - This is a texas Holdem poker hand that ranks high in poker order due to its relative strength compared to other starting hands. It’s a tricky hand to play and might require you to fold even after hitting a pair on the flop. If you miss the flop with this poker hand, it’s best to fold and stay out of trouble.
Also known as Little Slick, Antony & Cleopatra, Mrs Slick, Rocket Queen.
8. Ace-King Offsuit - This is the best poker hand to be calling in position pre-flop to keep the pot small and also get paid if you pair one of the two hole cards. It’s not as strong as its suited counterpart and you have to keep in mind that you’ve reduced your chances of hitting a flush, however, it’s still 40% favourite.
9. Ace-Jack Suited - The potential of this hand like an AK or AQ suited are the odds of hitting a royal flush. However, this texas Holdem poker hand needs to be played wisely especially when you have a player raising in early position. An AK or AQ will have you beat and it’s wise to not fall in love with your AJ suited.
Also known as Hijack, Jackass, Apple Jacks.
10. King-Queen Suited - King-queen suited is a great texas Holdem poker hand that is known to flop well. It opens up a large number of straights and flushes, and the odds of hitting a high pair making it one of the best poker hands to play.
Also known as Marriage.
Poker Card Rankings Explained
Royal Flush - A♥ K♥ Q♥ J♥ 10♥
Straight Flush - Q♥ J♥ 10♥ 9♥ 8♥
Four of a kind - 3♣ 3♠ 3♦ 3♥ 8♥
Full House - Q♦ Q♠ Q♣ 2♣ 2♦
Flush - 5♦ J♦ 3♦ K♦ 4♦
Straight - 5♣ 4♠ 3♠ 2♥ A♦
Three of a kind or set - 8♦ 8♠ 8♣ K♠ A♠
Two Pair - 9♠ 9♥ 5♦ 5♥ A♠
One Pair - 6♦ 6♥ 2♥ 5♠ K♣
High Card - Q♠ 5♣ 4♦ 3♦ 2♣
Poker Hand FAQ
Question 1: What is the best poker hand?
Question 2: What is the worst starting hand in Texas Hold’em Poker Hands?
Question 3: In which order are the poker hands ranked?
Question 4: How are poker hands constructed?
Gameplay
Poker Variations
Poker Rules
Poker Tournaments
Poker Strategy
Poker Accessories
This post works with 5-card Poker hands drawn from a standard deck of 52 cards. The discussion is mostly mathematical, using the Poker hands to illustrate counting techniques and calculation of probabilities
Working with poker hands is an excellent way to illustrate the counting techniques covered previously in this blog – multiplication principle, permutation and combination (also covered here). There are 2,598,960 many possible 5-card Poker hands. Thus the probability of obtaining any one specific hand is 1 in 2,598,960 (roughly 1 in 2.6 million). The probability of obtaining a given type of hands (e.g. three of a kind) is the number of possible hands for that type over 2,598,960. Thus this is primarily a counting exercise.
___________________________________________________________________________
Preliminary Calculation
Usually the order in which the cards are dealt is not important (except in the case of stud poker). Thus the following three examples point to the same poker hand. The only difference is the order in which the cards are dealt.
These are the same hand. Order is not important.
The number of possible 5-card poker hands would then be the same as the number of 5-element subsets of 52 objects. The following is the total number of 5-card poker hands drawn from a standard deck of 52 cards.
The notation is called the binomial coefficient and is pronounced “n choose r”, which is identical to the number of -element subsets of a set with objects. Other notations for are , and . Many calculators have a function for . Of course the calculation can also be done by definition by first calculating factorials.
Thus the probability of obtaining a specific hand (say, 2, 6, 10, K, A, all diamond) would be 1 in 2,598,960. If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of all diamond cards? It is
This is definitely a very rare event (less than 0.05% chance of happening). The numerator 1,287 is the number of hands consisting of all diamond cards, which is obtained by the following calculation.
The reasoning for the above calculation is that to draw a 5-card hand consisting of all diamond, we are drawing 5 cards from the 13 diamond cards and drawing zero cards from the other 39 cards. Since (there is only one way to draw nothing), is the number of hands with all diamonds.
If 5 cards are randomly drawn, what is the probability of getting a 5-card hand consisting of cards in one suit? The probability of getting all 5 cards in another suit (say heart) would also be 1287/2598960. So we have the following derivation.
Thus getting a hand with all cards in one suit is 4 times more likely than getting one with all diamond, but is still a rare event (with about a 0.2% chance of happening). Some of the higher ranked poker hands are in one suit but with additional strict requirements. They will be further discussed below.
Another example. What is the probability of obtaining a hand that has 3 diamonds and 2 hearts? The answer is 22308/2598960 = 0.008583433. The number of “3 diamond, 2 heart” hands is calculated as follows:
One theme that emerges is that the multiplication principle is behind the numerator of a poker hand probability. For example, we can think of the process to get a 5-card hand with 3 diamonds and 2 hearts in three steps. The first is to draw 3 cards from the 13 diamond cards, the second is to draw 2 cards from the 13 heart cards, and the third is to draw zero from the remaining 26 cards. The third step can be omitted since the number of ways of choosing zero is 1. In any case, the number of possible ways to carry out that 2-step (or 3-step) process is to multiply all the possibilities together.
___________________________________________________________________________
The Poker Hands
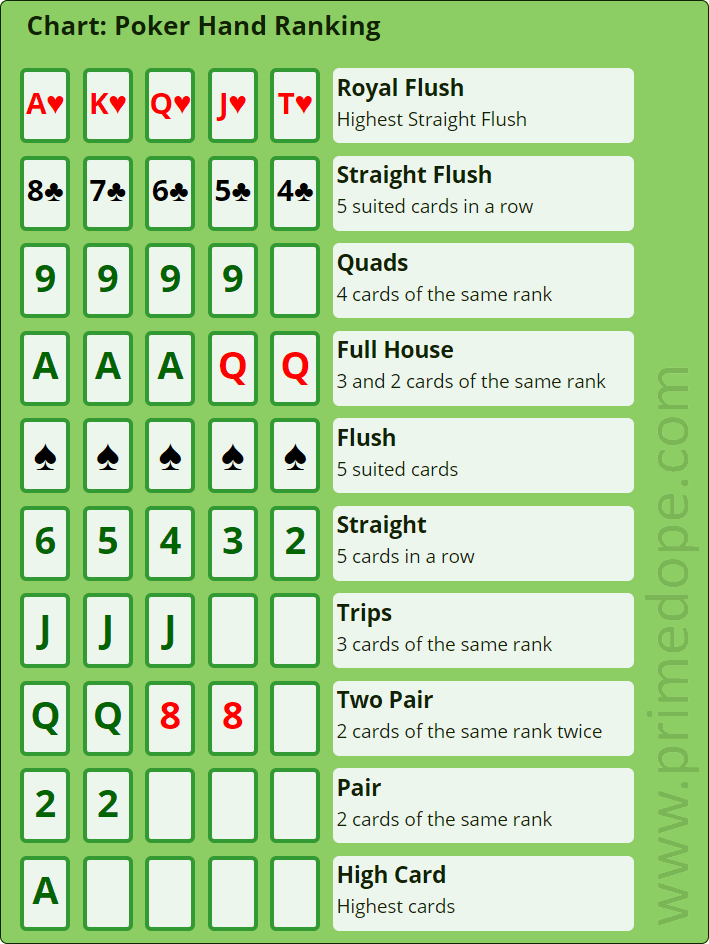
Here’s a ranking chart of the Poker hands.
The chart lists the rankings with an example for each ranking. The examples are a good reminder of the definitions. The highest ranking of them all is the royal flush, which consists of 5 consecutive cards in one suit with the highest card being Ace. There is only one such hand in each suit. Thus the chance for getting a royal flush is 4 in 2,598,960.
Royal flush is a specific example of a straight flush, which consists of 5 consecutive cards in one suit. There are 10 such hands in one suit. So there are 40 hands for straight flush in total. A flush is a hand with 5 cards in the same suit but not in consecutive order (or not in sequence). Thus the requirement for flush is considerably more relaxed than a straight flush. A straight is like a straight flush in that the 5 cards are in sequence but the 5 cards in a straight are not of the same suit. For a more in depth discussion on Poker hands, see the Wikipedia entry on Poker hands.

The counting for some of these hands is done in the next section. The definition of the hands can be inferred from the above chart. For the sake of completeness, the following table lists out the definition.
Definitions of Poker Hands
| Poker Hand | Definition | |
|---|---|---|
| 1 | Royal Flush | A, K, Q, J, 10, all in the same suit |
| 2 | Straight Flush | Five consecutive cards, |
| all in the same suit | ||
| 3 | Four of a Kind | Four cards of the same rank, |
| one card of another rank | ||
| 4 | Full House | Three of a kind with a pair |
| 5 | Flush | Five cards of the same suit, |
| not in consecutive order | ||
| 6 | Straight | Five consecutive cards, |
| not of the same suit | ||
| 7 | Three of a Kind | Three cards of the same rank, |
| 2 cards of two other ranks | ||
| 8 | Two Pair | Two cards of the same rank, |
| two cards of another rank, | ||
| one card of a third rank | ||
| 9 | One Pair | Three cards of the same rank, |
| 3 cards of three other ranks | ||
| 10 | High Card | If no one has any of the above hands, |
| the player with the highest card wins |
Poker Card Combinations Ranking
___________________________________________________________________________
Counting Poker Hands
Straight Flush
Counting from A-K-Q-J-10, K-Q-J-10-9, Q-J-10-9-8, …, 6-5-4-3-2 to 5-4-3-2-A, there are 10 hands that are in sequence in a given suit. So there are 40 straight flush hands all together.
Four of a Kind
There is only one way to have a four of a kind for a given rank. The fifth card can be any one of the remaining 48 cards. Thus there are 48 possibilities of a four of a kind in one rank. Thus there are 13 x 48 = 624 many four of a kind in total.
Full House
Let’s fix two ranks, say 2 and 8. How many ways can we have three of 2 and two of 8? We are choosing 3 cards out of the four 2’s and choosing 2 cards out of the four 8’s. That would be = 4 x 6 = 24. But the two ranks can be other ranks too. How many ways can we pick two ranks out of 13? That would be 13 x 12 = 156. So the total number of possibilities for Full House is
Note that the multiplication principle is at work here. When we pick two ranks, the number of ways is 13 x 12 = 156. Why did we not use = 78?
Flush
There are = 1,287 possible hands with all cards in the same suit. Recall that there are only 10 straight flush on a given suit. Thus of all the 5-card hands with all cards in a given suit, there are 1,287-10 = 1,277 hands that are not straight flush. Thus the total number of flush hands is 4 x 1277 = 5,108.
Straight
There are 10 five-consecutive sequences in 13 cards (as shown in the explanation for straight flush in this section). In each such sequence, there are 4 choices for each card (one for each suit). Thus the number of 5-card hands with 5 cards in sequence is . Then we need to subtract the number of straight flushes (40) from this number. Thus the number of straight is 10240 – 10 = 10,200.
Three of a Kind
There are 13 ranks (from A, K, …, to 2). We choose one of them to have 3 cards in that rank and two other ranks to have one card in each of those ranks. The following derivation reflects all the choosing in this process.
Two Pair and One Pair
These two are left as exercises.
Poker Combinations Rankings
High Card
The count is the complement that makes up 2,598,960.
The following table gives the counts of all the poker hands. The probability is the fraction of the 2,598,960 hands that meet the requirement of the type of hands in question. Note that royal flush is not listed. This is because it is included in the count for straight flush. Royal flush is omitted so that he counts add up to 2,598,960.
Poker Pairs Ranking
Probabilities of Poker Hands
Poker-hand-ranking-combinations
| Poker Hand | Count | Probability | |
|---|---|---|---|
| 2 | Straight Flush | 40 | 0.0000154 |
| 3 | Four of a Kind | 624 | 0.0002401 |
| 4 | Full House | 3,744 | 0.0014406 |
| 5 | Flush | 5,108 | 0.0019654 |
| 6 | Straight | 10,200 | 0.0039246 |
| 7 | Three of a Kind | 54,912 | 0.0211285 |
| 8 | Two Pair | 123,552 | 0.0475390 |
| 9 | One Pair | 1,098,240 | 0.4225690 |
| 10 | High Card | 1,302,540 | 0.5011774 |
| Total | 2,598,960 | 1.0000000 |
Poker Combinations Rank
___________________________________________________________________________
2017 – Dan Ma